Introduction:
The study of motion has always been a cornerstone of physics, with concepts like force, mass, and acceleration forming the foundation of this field. Among the most intriguing topics in dynamics is the acceleration with variable force equation, which describes how objects respond to non-constant forces. Unlike scenarios involving constant forces, where acceleration remains steady, this equation provides a framework for understanding more complex situations where force changes over time or space.
The Basics of Acceleration and Force
Before discussing the specifics of the acceleration with the variable force equation, it is essential to revisit the fundamental relationship between force and acceleration. Isaac Newton’s second law of motion states that the force acting on an object is equal to the product of its mass and acceleration. This law applies universally but is often simplified under the assumption of constant force.
However, in many real-world scenarios, forces vary. Examples include the thrust of a rocket engine changing during ascent, aerodynamic drag increasing with velocity, or the force exerted by a spring as it compresses or extends. In such cases, the acceleration with variable force equation becomes a critical tool for analysis.
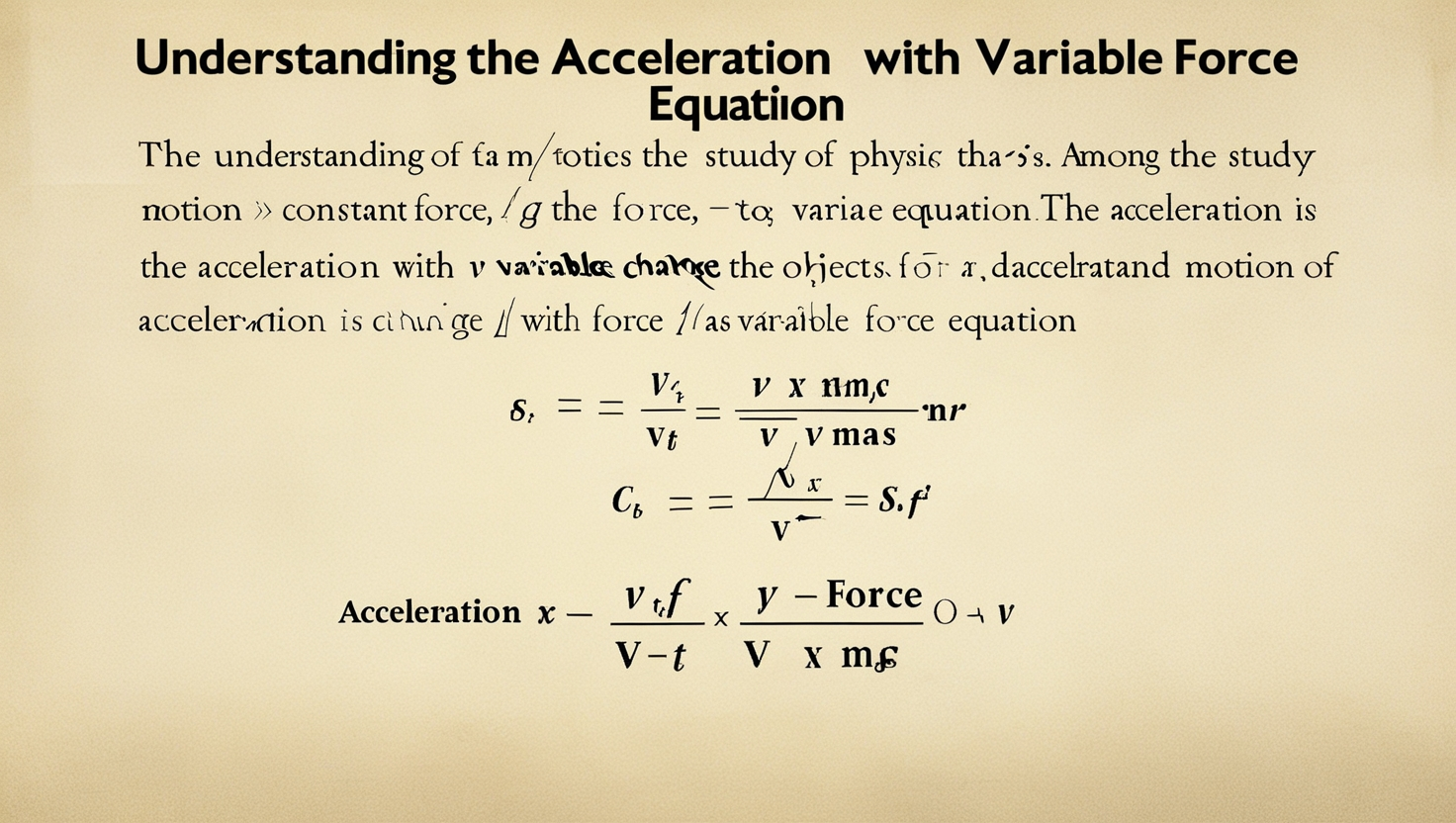
Deriving the Acceleration with Variable Force Equation
To derive the acceleration with variable force equation, one must consider Newton’s second law in a more general form. When the force is a function of time r varies with position, the relationship becomes:
- or is the instantaneous acceleration,
- or is the variable force,
- is the mass of the object.
This generalized approach to acceleration with variable force equations allows physicists to model systems with non-uniform forces, enabling predictions about motion under varying conditions.
Applications of the Acceleration with Variable Force Equation
The acceleration with variable force equation finds application in numerous fields of science and engineering. A few prominent examples include:
- Projectile Motion with Air Resistance: Airn classical projectile motion. In classical projectile motion, however, resistance—a variable force dependent on velocity—must be included in inaccurate predictions. Here, the acceleration with variable force equation provides insight into the projectile’s trajectory.
- Oscillatory Systems: In systems involving springs, the restoring force obeys Hooke’s law, where is the spring constant and is the displacement. This variable force changes acceleration as the spring compresses or stretches.
- Rocket Propulsion: During a rocket’s ascent, the thrust produced by its engines varies due to changes in fuel flow and atmospheric conditions. Using the acceleration with variable force equation helps engineers design efficient trajectories.
- Gravitational Dynamics: The gravitational force varies with distance when gravitational force variess with distance when an object moves within a nonon-uniforgravitationhe acceleration with variable force equation becomes essential for precise calculations.
Mathematical Analysis of the Equation
An analysis under the acceleration with variable force equation often requires integration or differentiation to account for the changing nature of force. For instance:
- Time-Dependent Forces: When known, acceleration can be integrated to find velocity and position over time. This process involves solving differential equations, such as:
- Position-Dependent Forces: Withenvaries with position, the work-energy principle often complements the acceleration with variable force equation. The total work done on the object relates to its change in kinetic energy:
- Numerical Solutions: In complex cases where analytical solutions are not feasible, numerical methods like Euler’s or Runge-Kutta algorithms help solve the equations derived from the acceleration with variable force equation.
Challenges in Applying the Equation
Despite its versatility, the acceleration with variable force equation poses challenges. Accurately modelling variable forces requires precise knowledge of how these forces behave. In practical scenarios, forces may depend on multiple factors, such as velocity, position, and time, making the system highly nonlinear. Additionally, solving the resulting equations often demands computational resources and expertise.
For instance, in fluid dynamics, drag force depends on factors like fluid density, object shape, and velocity squared. Incorporating these complexities into the acceleration with variable force equation involves advanced modelling and simulation techniques.
Experimental Validation of the Equation
Experimental physics crucially in validating the acceleration with variable force equation. By measuring motion under controlled conditions, researchers confirm the accuracy of theoretical predictions. For example:
- In pendulum experiments, the variable tension in the string and gravitational force create a scenario where acceleration changes continuously.
- In collision studies, forces vary rapidly during impact, providing a dynamic testbed for the equation.
These experiments underscore the importance of the acceleration with variable force equation in bridging theoretical physics and real-world observations.
Broader Implications of the Equation
Beyond its scientific applications, the acceleration with variable force equation influences technology and industry. Engineers leverage this equation to design safer vehicles, optimise energy usage in machines, and predict the behaviour of materials under stress. In astrophysics, it aids in understanding celestial mechanics and space exploration missions.
Furthermore, the equation has educational value. It illustrates the beauty of mathematics and physics in describing complex systems. Studying it encourages critical thinking, problem-solving, and appreciation for the interconnectedness of natural phenomena.
Conclusion: The Enduring Significance of the Acceleration with Variable Force Equation
The acceleration with variable force equation represents a cornerstone of modern physics, capturing the dynamics of motion in the presence of non-constant forces. Its derivation, applications, and challenges highlight the depth and complexity of this fundamental concept. From explaining natural phenomena to driving technological innovation, this equation exemplifies the power of mathematical modelling in understanding our world.
As researchers and engineers continue to push the boundaries of science, the acceleration with variable force equation will remain a vital tool, shaping our comprehension of motion and its governing forces. Its study enriches our knowledge and inspires new generations to explore the dynamic universe around us.